Magic Squares

I hope you enjoy these examples of a variety of magic squares.

This section of my site consists mostly of examples, with a minimum of explanation and theory.
Refer to the other two sections for Magic Stars and Number Patterns.
This site should be of interest to middle and high school students and teachers, and anyone interested in recreational mathematics.

Magic Squares are a form of number pattern that has been around for thousands of years.

For a pure or normal magic square, all rows, columns, and the two main diagonals must sum to the same value and the numbers used must be consecutive from 1 to n², where n is the order of the square.
Many variations exist that contain numerous other features.

I show on these pages samples of the large variety of magic squares. My descussions will be limited to brief comments on the individual illustrations. Perhaps in the future, I will add more in depth information in the way of history, theory, construction methods, etc.

Acknowledgments: As with all the mateial on this site, most of these illustrations are original with myself or I consider them in the public domain (i.e. I have multiple sources for the illustration). Many of the more unusual figures are one of a kind and I so acknowledge the author with thanks for permission to use them.

Contents

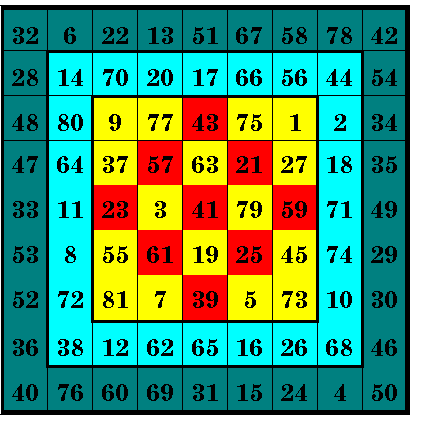
Set of Orders 3, 4, and 5

Three simple magic squares together use the numbers from 1 to 50. None of the three is a pure magic square because none uses consecutive numbers starting at 1. S₃ = 42, S₄ = 91, S₅ = 157.

Orders 3, 5, 7, 9 Inlaid

John R. Hendrick's inlaid magic squares

An order-9 magic square with three inlaid magic squares of Orders 3, 5, and 7. The order-3 is rotated 45 degrees and is referred to as a diamond inlay. Note that the smaller and larger numbers are mixed throughout the square, not in the outside border as they would be with a bordered magic square.
These outside rings are called expansion bands to diferentiate them from the borders (of a bordered or concentric magic square), which have 2n+2 low and high numbers in the border .

S₃ = 123, S₅ = 205, S₇ = 287, S₉ = 369. Numbers used are 1 to 81, so Order-9 is a pure magic square.

Order-20 with 4 Inlays

J.R.Hendricks, Magic square course (self-published) pp290-294
I assembled this from a boilerplate design by John Hendricks. He provides the frame, and four of each of the order-7 inlays,
one for each quadrant. It is then simply a matter of deciding which type of inlay to put in each quadrant.

The order-7 (upper right corner) is a pandiagonal so may be altered by shifting rows or columns.
The order-5 (lower left quadrant) is also a pandiagonal.
The order-20, because it contains the consecutive numbers from 1 to 400, is a pure magic square
Magic sums are: U.L. 1477, 1055, 633; -- U.R. 1337; -- L.L. 1470, 1050;  -- L. R. 1330, 950, 570

Four plus five equals nine

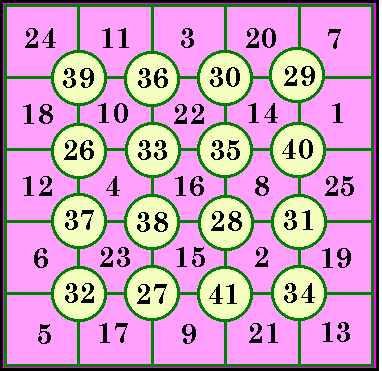
Numbers 1 to 25 arranged as an order-5 pandiagonal pure magic square.

Numbers 26 to 41 arranged as an embedded order-4 pandiagonal magic square.

Together, they make an order-9 magic square. Any one of the rows and any one of the columns of the order-4 is counted twice.

S₄ = 134, S₅ = 65, S₉ = 199

If we use the series from 70 to 110 instead of 1 to 41, the magic constant of both order-4 and order-5 is 410 !

As far as I can determine, this type of magic square originated with Kenneth Kelsey of Great Britain.

Order-18 based on 1/19

The full term decimal expansion of the prime number 19 when multiplied by the values 1 to 18, may be arranged in a simple magic square of order-18, if the decimal point is ignored. All 18 rows, columns and the two main diagonals sum to the same value. S = 81. Of course this is not a pure magic square because a consecutive series of numbers from 1 to n is not used.
Point of interest: 81 is also a cyclic number (of period 9). 1/81 = .0123456790123456 ... . Only the 8 is missing. Too bad!

This magic square was designed by Harry A. Sayles and published in the Monist before 1916.
W. S. Andrews, Magic Squares and Cubes, Dover Publ., 1917, p.176

The next cyclic number (in base 10) that is capable of forming a magic square in this fashion, is n/383.
In an e-mail dated July 20/01, Simon Whitechapel pointed out that many such magic squares may be formed using full period cyclic numbers in other bases.

Below we show that the numbers n/19 can be multiplied simply by shifting left. Obviously, each row and column add to the same value (a property of all such lists).

 1/19 = .0   5   2   6   3   1   5   7   8   9   4   7   3   6   8   4   2   1
10/19 = .5   2   6   3   1   5   7   8   9   4   7   3   6   8   4   2   1   0
 5/19 = .2   6   3   1   5   7   8   9   4   7   3   6   8   4   2   1   0   5
12/19 = .6   3   1   5   7   8   9   4   7   3   6   8   4   2   1   0   5   2
 6/19 = .3   1   5   7   8   9   4   7   3   6   8   4   2   1   0   5   2   6
 3/19 = .1   5   7   8   9   4   7   3   6   8   4   2   1   0   5   2   6   3
11/19 = .5   7   8   9   4   7   3   6   8   4   2   1   0   5   2   6   3   1
15/19 = .7   8   9   4   7   3   6   8   4   2   1   0   5   2   6   3   1   5
17/19 = .8   9   4   7   3   6   8   4   2   1   0   5   2   6   3   1   5   7
18/19 = .9   4   7   3   6   8   4   2   1   0   5   2   6   3   1   5   7   8
 9/19 =  4   7   3   6   8   4   2   1   0   5   2   6   3   1   5   7   8   9
14/19 = .7   3   6   8   4   2   1   0   5   2   6   3   1   5   7   8   9   4
 7/19 = .3   6   8   4   2   1   0   5   2   6   3   1   5   7   8   9   4   7
13/19 = .6   8   4   2   1   0   5   2   6   3   1   5   7   8   9   4   7   3
16/19 = .8   4   2   1   0   5   2   6   3   1   5   7   8   9   4   7   3   6
 8/19 = .4   2   1   0   5   2   6   3   1   5   7   8   9   4   7   3   6   8
 4/19 = .2   1   0   5   2   6   3   1   5   7   8   9   4   7   3   6   8   4
 2/19 = .1   0   5   2   6   3   1   5   7   8   9   4   7   3   6   8   4   2